
| Biologie | Botanica | Chimie | Didactica | Fizica | Geografie |
| Gradinita | Literatura | Matematica |
Matematica
|
|
Qdidactic » didactica & scoala » matematica Homographic Function and its Application to the Study of Recursion Sequences |
Homographic Function and its Application to the Study of Recursion Sequences
Homographic Function and its Application to the Study of Recursion Sequences
This article gives an overall view of homographic function and a summary of its properties, as well as
few of its applications respectively recursion sequences.
Many problems with a difficult level of difficulty, encountered especially at the national and international mathematics competisions, deal with the sequences defined by the recursion formula:
(1) 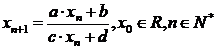 and
and ![]()
If the sequence ![]() is given by
is given by ![]() , where
, where ![]() ,
,
and if ![]() defined by (1) is
written as
defined by (1) is
written as ![]() ,
,
then, ![]() ,
,
and if ![]() , then
, then ![]() .
.
Since ![]() is a discontinuous
function, so is
is a discontinuous
function, so is ![]() .
.
But, discontinuous
functions are not derivable. It follows
that one cannot use derivatives and their properties to study ![]() .
.
However, a possible method
to study ![]() is to extend
is to extend ![]() to a new domain
to a new domain 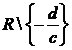 so that the function
is continuous.
so that the function
is continuous.
Therefore,
(2) 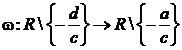 ,
,
and this new function, ![]() is called the
homographic function.
is called the
homographic function.
This way, the sequences defined by (1) can be studyed easier. More than that, this method can be generalized, giving rise to new problems studied by calculus.
Let us consider first some of the properties of the homographic function.
Using these properties one can solve in a more concise and ellegantly way the sequences in (1).
1. Some of the Properties of the Homographic Function
The homographic function is a basic concept in Geometry through its geometric aspect called homographic transformation.
In Algebra, the homographic transformations body forms a group relative to the composition of functions.
Let us consider the homographic function:
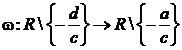 ,
, ![]() , and
, and ![]() ,
, ![]() .
.
The following are its properties:
P1. The homographic function is bijection;
P2.
The point 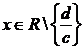 is a fixed point if
is a fixed point if ![]() is the solution of
is the solution of ![]() ;
;
P3. If
![]() the homographic function is strictly increasing;
the homographic function is strictly increasing;
If ![]() the homographic
function is strictly decreasing;
the homographic
function is strictly decreasing;
P4.
The homographic function is involutory (![]() ) if and only if
) if and only if ![]() ;
;
P5. If
the homographic function (2) defined and continuous on the interval ![]() is strictly increasing
on
is strictly increasing
on ![]() , then the sequence
, then the sequence ![]() for which
for which ![]() and (1)
and (1) 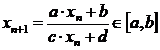 is:
is:
a) increasing, if ![]() ;
;
b) decreasing, if![]() .
.
The bijection extension ![]() of the homographic function
of the homographic function ![]() is:
is:
(3) ![]() ,
, 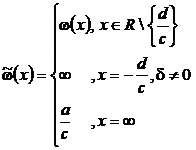
2. Associated Homographic Function ![]() of Homography
of Homography ![]() Between Two Sets of
Points with the Same Support
Between Two Sets of
Points with the Same Support
Let us consider the sets of points (M) and (M') on the same line ("d").
On the Cartesian system ![]() , where
, where![]() is the x
coordinate of point M.
is the x
coordinate of point M.
Let M and M' be the points that generate the sets of points (M) and (M') and being on the same line d.
Let us use the notation![]() and
and ![]() .
.
As we saw, the point ![]() does not have an image
through the homographic function
does not have an image
through the homographic function![]() , and, at the
, and, at the
same time, there is no ![]() so that
so that ![]() .
.
Considering
(3), conveniently, we can attach to the line d a new point ![]() , called
, called ![]() , in the set of points (M) and
, in the set of points (M) and ![]() in the sets of points
(M') respectively, so that: ,
in the sets of points
(M') respectively, so that: ,
a)
![]() is the image of point
is the image of point ![]() with the x coordinate
with the x coordinate ![]() , that is
, that is 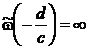 ;
;
b)
![]() is the image of point
is the image of point ![]() with the x
coordinate
with the x
coordinate ![]() , that is
, that is ![]() .
.
This way one obtained an
enlarged line, or projective line ![]() .
.
![]() represents the point
at infinity, or the improper point on
line d.
represents the point
at infinity, or the improper point on
line d.
Definition.
An homographic transformation, or homography of a projective line ![]() is the function
is the function
![]() , where
, where ![]() .
.
Notes
a) the homography ![]() is associated to the
extension
is associated to the
extension ![]() of the homographic
function
of the homographic
function ![]() , and vice versa.
, and vice versa.
b) as we saw, ![]() and
and ![]() , where
, where ![]() and
and ![]() .
.
c) points L and L' are called the limit points for ![]() .
.
d) one does not uses different notations for the
extension ![]() of the homographic
function
of the homographic
function
![]() or for the associated homographic
transformation. Depending on the context
or for the associated homographic
transformation. Depending on the context ![]() can be either the
homographic function or the associated homographic transformation. In the same
manner, a point is either a real number
can be either the
homographic function or the associated homographic transformation. In the same
manner, a point is either a real number ![]() (including
(including ![]() ) or the point
) or the point ![]() .
.
Properties of ![]() .
.
|
P1. A
homograpfy between two sets of points d=(M) and d'=(M') on the same ![]() or on a different one,
or on a different one,
is determined by three pairs of points.
P2. A
homograpfy ![]() between two sets of
points d=(M) and d'=(M') on the same
between two sets of
points d=(M) and d'=(M') on the same ![]() or on a different one,
uses the same ratio, that is
or on a different one,
uses the same ratio, that is ![]() , where
, where ![]()
P3.
Homographies of projective line ![]() form a group.
form a group.
3. Double Points of a Homography ![]() , between the Two Sets
of Points Superimposed
, between the Two Sets
of Points Superimposed
Let us consider two sets
of points ![]() and
and ![]() (where the two sets of
points are superimposed, but have unique individuality with regard to the
homography
(where the two sets of
points are superimposed, but have unique individuality with regard to the
homography ![]() .
.
Let
(4) ![]() given by (3).
given by (3).
There are the following situations;
a) double points (united)
b) involutory homographies or the involutory
projective line ![]()
Definition: Point ![]() is called double point or unit point of homography (4) between two sets of points
superimposed if and only if
is called double point or unit point of homography (4) between two sets of points
superimposed if and only if ![]() .
.
Note:
If ![]() is the equation for
is the equation for ![]() in
in ![]() , then
, then ![]()
and ![]() .
.
Therefore:
a) If ![]() the homografy
the homografy ![]() has two double
distinct points
has two double
distinct points ![]() with x-coordinates
with x-coordinates ![]() . the homography is
called general homographie.
. the homography is
called general homographie.
b) If ![]() the homografie
the homografie ![]() is called parabolic
homographie.
is called parabolic
homographie.
Theorem:
Ratio formed by the pair of the distinct double points ![]() and any other pair of
points
and any other pair of
points ![]() is constant and
written as
is constant and
written as ![]()
Note: according to the previous theorem,
a) (5) ![]() .
.
This expression is called
the canonic form of the general homography ![]()
b) considering (5), one
can write (6)![]() , the equation of the general homography between the two sets
of points in terms of
, the equation of the general homography between the two sets
of points in terms of ![]() and
and ![]() .
.
c) considering (6) and P3 (in paragraph 1) gives a
general homography (![]() ) is strictly increasing if
) is strictly increasing if ![]() , and strictly decreasing if
, and strictly decreasing if ![]()
4. Classifications of Homographies.
The Characteristic Equation of a Homography.
Canonic Form of a Parabolic Homography (![]() ).
).
Let us consider a
homography between the two sets of points with the same support(superimposed)
given by (2) or (3). Then, the fixed
points of the homographic application ![]() or
or ![]() is given by the
equation:
is given by the
equation:
(7) ![]() .
.
So, ![]() and
and ![]() .
.
The discriminant in equation (7) is:
(8) ![]() , where
, where ![]() .
.
Considering all the above, homographies are classified as following:
A.
If ![]() then,
then, ![]() , and the homography is called hyperbolic.
, and the homography is called hyperbolic.
B.
If ![]() then,
then, ![]() , and the homography is called parabolic.
, and the homography is called parabolic.
C.
If ![]() then,
then, 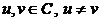 , and the homography is called elliptic.
, and the homography is called elliptic.
In the study of
homographies, a special importance is given to birapport invariant ![]() whose formula is
whose formula is
(9) 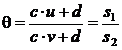 ;
; ![]() and
and![]() are in fact the roots of equation (10').
are in fact the roots of equation (10').
(10) 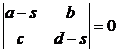 is called the
characteristic equation of the homography.
is called the
characteristic equation of the homography.
(10') ![]() is equivalent to (10).
is equivalent to (10).
Considering (5) and ![]() , it follows
, it follows
(11) ![]() . This equation is called the canonic form of the parabolic
homography.
. This equation is called the canonic form of the parabolic
homography.
Definition:
An homography ![]() between the points of
a line is called direct (indirect) if the associated homographic
function
between the points of
a line is called direct (indirect) if the associated homographic
function ![]() is increasing
(decreasing respectively).
is increasing
(decreasing respectively).
Based on the definition the following theorem is obvious.
Theorem:
If the homographis ![]() ,
, ![]() are indirect, then the
homography
are indirect, then the
homography ![]() is direct.
is direct.
5. The primitive Root of the binomial equation ![]() . Involutions
. Involutions
Let Cn
=![]() , where
, where  .
.
P1. (Cn, .) is commutative group, subgroup of group (C, .).
Definition: An element ![]() of group (G, .) has period
of group (G, .) has period ![]() if
if ![]() is the smallest
natural number, not zero, so that
is the smallest
natural number, not zero, so that ![]() .
.
Note:
a) The homographic function ![]() has a period
has a period ![]() if
if ![]() is the smallest natural number so that
is the smallest natural number so that
![]() ;
;
b) ![]() C is of a period
C is of a period ![]() or is a primitive root
of exponent
or is a primitive root
of exponent ![]() of the unit if
of the unit if ![]() , and
, and ![]() is the smallest
natural number with this property;
is the smallest
natural number with this property;
d) canonic form (5) of the general homography (![]() ) allows finding the period of a homography
) allows finding the period of a homography
e) ( and the associated homography) with the help of
the primitive roots of the binomial equation ![]() .
.
Definition: It is called the primitive root of binomial equation![]() , each root of the equation that is not a root of a
binomial equation with degree less than
, each root of the equation that is not a root of a
binomial equation with degree less than ![]() .
.
The roots of binomial equations have the following properties:
P1.
Each root of binomial equation ![]() is a root of
binomial equation
is a root of
binomial equation ![]() if
if ![]() divide
divide![]() .
.
P2. A
common root of binomial equations ![]() and
and ![]() is a root of
binomial equation
is a root of
binomial equation
![]() , where
, where![]() is the least common divisor of
is the least common divisor of ![]() and
and ![]() .
.
P3.
Primitive roots of binomial equation ![]() are
are![]() , where
, where![]() takes all prime values with
takes all prime values with ![]() but less than
but less than![]() .
.
P4. If
![]() is a primitive of
binomial equation
is a primitive of
binomial equation![]() , then the
roots of the equation are
, then the
roots of the equation are ![]() ,
, ![]() . In other words, the primitive root
. In other words, the primitive root ![]() of binomial equation
of binomial equation![]() is the generator element of group (Cn, .).
is the generator element of group (Cn, .).
P5.
infinite sequence of successive exponents of primitive roots ![]() of index
of index ![]() of binomial equation
of binomial equation ![]() is periodical, of
period
is periodical, of
period![]() .
.
P6.
one necessary and sufficient condition for the homographic function ![]() with double
distinctive points
with double
distinctive points ![]() and associative
periodic homography
and associative
periodic homography ![]() of period
of period ![]() , is:
, is: ![]() a primitive root of
index
a primitive root of
index ![]() in equation
in equation ![]() .
.
Using the canonic
form (5) and ![]() or
or ![]() , we have:
, we have:
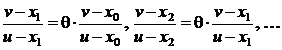 ,
,
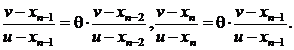
multiplying the relations and simplifying,
(12) 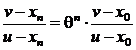 .
.
In (12), given the double distinctive points of
the homography, their order and invariable ratio ![]() , one can calculate
, one can calculate ![]() in terms of
in terms of ![]() :
:
(13) 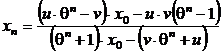 .
.
Homographic functions with
fixed coincident points (![]() ), and associated parabolic homographies cannot be
periodical, as you can notice in (11).
), and associated parabolic homographies cannot be
periodical, as you can notice in (11).
6. Involutive Homographic Function
Definition.
Let ![]() be a homography, a
corresponding pair of points
be a homography, a
corresponding pair of points ![]() is called an involutory pair, if simultaneously exist
is called an involutory pair, if simultaneously exist ![]() that is if
that is if ![]() .
.
If all pairs of a
homography ![]() are involutory, then
homography
are involutory, then
homography ![]() is called involutive homongraphy.
is called involutive homongraphy.
Note Involutory
homography is a periodic homography of period![]() .
.
Considering the definition and the note, we have the following property:
P1. A
necessary and sufficient condition for homography ![]() between two sets of
points superimposed
between two sets of
points superimposed
given by(3) to be involutory is : ![]() .
.
7. Examples.
Example 1)
Let the sequence defined
by the recursion formula be  and
and![]() .
.
Find ![]() .
.
Solution
Let us consider the
homographic function 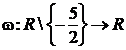 ,
, ![]() .
.
Notice that: ![]() .
.
This homography has fixed
points given by the equation ![]() .
.
Then, the homography is
hyperbolic, and ![]() and
and ![]() are fixed points.
are fixed points.
The characteristic
equation of this homography is: ![]() . Then,
. Then, ![]() . The invariant ratio associated to this homography is:
. The invariant ratio associated to this homography is: ![]() .
.
Using (12) and the above
data, one obtain: 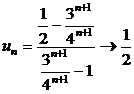 .
.
Example 2)
One sequence ![]() is defined by :
is defined by :  .
.
Show that the sequence is convergent and calculate its limit. (Mathematics Olympiad - Austria, 1979).
Solution
![]() . Since
. Since ![]() then
then ![]() is increasing, and because
is increasing, and because ![]() (using P5 din paragraph 1), then the
sequence
(using P5 din paragraph 1), then the
sequence ![]() is decreasing and is
inferior bounded by
is decreasing and is
inferior bounded by ![]() , that is convergent.
, that is convergent.
Taking the limit of the
recursion relation, one obtain![]() .
.
Example 3)
Let us consider the sequence ![]() ,
, ![]() , and
, and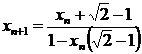 ,
, ![]() . Show that the sequence is periodic and determine the
period. (tie 1972 - Liviu Pirsan).
. Show that the sequence is periodic and determine the
period. (tie 1972 - Liviu Pirsan).
Solution
Let us consider the
homographic function: 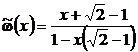 . Notice that
. Notice that ![]() . The double points of the homography are the roots of the
equation
. The double points of the homography are the roots of the
equation ![]() . Then,
. Then, ![]() The invariant ratio of the elliptic homography
The invariant ratio of the elliptic homography ![]() is
is ![]() , that is
, that is ![]() .
.
Since the smallest natural
number ![]() for which
for which ![]() is
is ![]() , is the primitive root of the unit. 3.
, is the primitive root of the unit. 3.
According to P6 (5) homographic function ![]() and its restriction
the sequence is periodic of period
and its restriction
the sequence is periodic of period![]() .
.
Example 4)
Determine one necessary
and sufficient condition for the sequence ![]() defined recurrently by
the relation
defined recurrently by
the relation 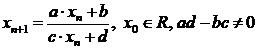 so that it is :
so that it is :
a) periodic, of period![]() ;
;
b) periodic, of period ![]() .
.
Solution
Let us consider the
homographic function ![]() . The fixed points of this homographic application are the
roots of the equation:
. The fixed points of this homographic application are the
roots of the equation:
(1) ![]() .
.
The invariant ratio ![]() of the general
homography (
of the general
homography (![]() ) is given by the relation:
) is given by the relation:
(2) ![]() .
.
For the homography ![]() with the double
distinct points (
with the double
distinct points (![]() ) be periodic of period
) be periodic of period ![]() , it is necessary for
, it is necessary for ![]() be a primitive root of
index
be a primitive root of
index ![]() in
in ![]() (this is a necessary
and sufficient condition ).
(this is a necessary
and sufficient condition ).
a) ![]() means
means ![]() ,
, ![]() . Considering (2) this
equality becomes:
. Considering (2) this
equality becomes:
(3) 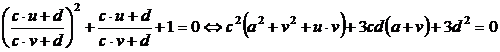 .
.
Applying Vičte's equations to (1) ,(![]() ) and substituting in (3) one obtain:
) and substituting in (3) one obtain:
(4) ![]() .
.
So (4) is a necessary and
sufficient condition for the sequence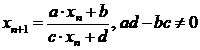 be periodic of period
be periodic of period ![]() .
.
b) ![]() implies
implies ![]() (
(![]() )
) ![]() (5). Considering
(5). Considering  where
where ![]() and
and ![]() are the roots of the
equation
are the roots of the
equation ![]() (6),
(6), ![]() .
.
Substituting ![]() in equation (5), one
obtain (7)
in equation (5), one
obtain (7) ![]() .
.
Applying Vičte's equations to (6), one obtain:
(8) ![]() .
.
Considering (7) si (8) one obtain:
(9) ![]() .
.
So (9) represents a
necessary and sufficient condition for the sequence 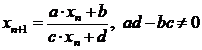 be periodic of period
be periodic of period ![]() .
.
Example 5)
Let us consider the
sequence defined by 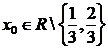 and the recursion
relation:
and the recursion
relation: 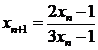 .
.
Show that the sequence is
periodic, of period![]() , and calculate the sum:
, and calculate the sum: ![]() .
.
Solution
Let us consider the
general homogaphy ![]() , where
, where ![]() . Notice that
. Notice that ![]() .
.
Considering 4a) and noticing that:
![]() .
.
Then the sequence defined
recurrently is periodic and of period ![]() .
.
Then: ![]() .
.
But, 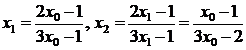 .
.
Then, 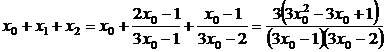 .
.
Note. Similarly to ex 4), the necessary and sufficient
condition for the sequence defined recurrently by the relation  , to be of period
, to be of period ![]() is:
is: ![]() .
.
Bibliography
- D.M. Batinetu: "Siruri", Ed. Albatros, Bucuresti, 1979;
- C. Avadanei, N. Avadanei, C. Boros, C. Ciuca: "De la matematica elementara spre matematica superioara", Ed. Academiei R.S.R., Bucuresti, 1987.
| Contact |- ia legatura cu noi -| | |
| Adauga document |- pune-ti documente online -| | |
| Termeni & conditii de utilizare |- politica de cookies si de confidentialitate -| | |
| Copyright © |- 2025 - Toate drepturile rezervate -| |
|
|
|||
|
|||
|
|||
Referate pe aceeasi tema | |||
|
| |||
|
|||
|
|
|||








